Chapter 1: Introduction to Rock-Paper-Scissors

The game of Rock-Paper-Scissors might seem simple and playful, but its connection to science is quite profound. Specifically, game theory, a branch of mathematics that examines strategic interactions, plays a crucial role in understanding the game. Researchers have explored effective strategies for winning Rock-Paper-Scissors, leading to fascinating insights that might surprise you.

Section 1.1: Understanding the Game Mechanics

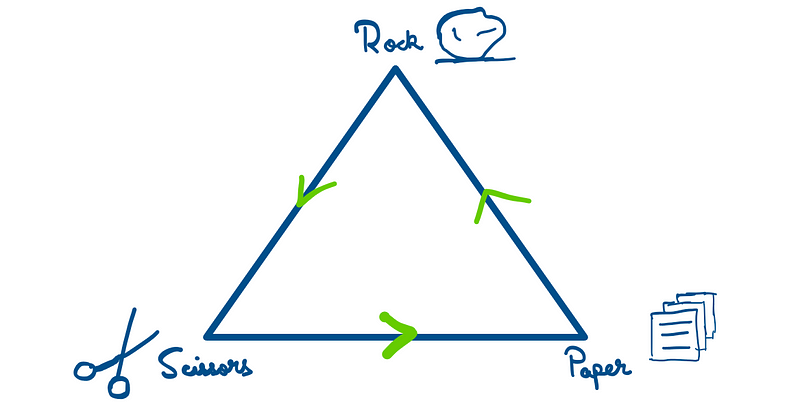
Before diving deeper, let's revisit the fundamental rules of Rock-Paper-Scissors. The game involves two participants and three choices: rock, paper, and scissors. Each player simultaneously selects one of the three options, and the winner is determined based on the following relationships: rock crushes scissors, scissors cut paper, and paper envelops rock. The winner is typically the one who secures the most victories in a set of rounds.

Chapter 2: Insights from Experimental Research

To address the discrepancies between theory and actual behavior, a study conducted in China with 360 participants sought to uncover patterns in gameplay. Participants were divided into groups of six and engaged in multiple rounds of Rock-Paper-Scissors.

The first video titled "HOW TO WIN ROCK PAPER SCISSORS!! // EASY TUTORIAL" provides practical tips on mastering the game and highlights the psychological aspects that influence decision-making.

The researchers identified two key patterns that contradicted the notion of chance:

1. Winners tended to repeat their winning choice in subsequent rounds.
2. Losers often opted to avoid the losing choice in the next round.

For example, if a player wins with 'Rock', they are likely to choose 'Rock' again in the following round. Conversely, if they lose with 'Rock', they tend to avoid it in the next round. This behavior reflects the psychological tendencies of players, which we will explore further.

The second video, "The Science of Rock, Paper, Scissors," delves into the psychological and strategic elements that influence player choices and outcomes in the game.

Section 2.1: Developing a Winning Strategy

Based on their findings, researchers proposed two conditional strategies, or heuristics, that proved more effective than the traditional game theory approach:

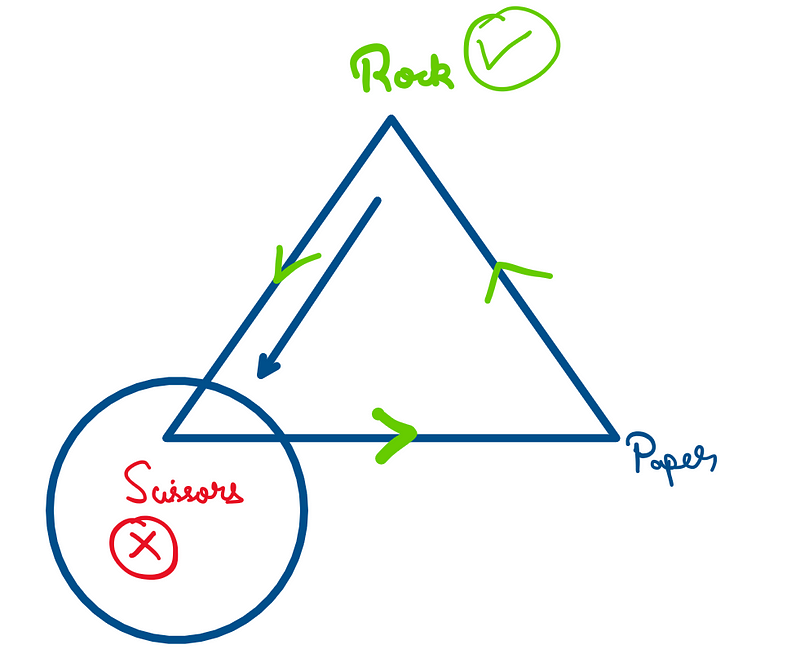
1. If you win with one choice, select the option your opponent just lost to in the following round.
2. If you lose with one choice, choose an option that neither player selected in the preceding round.

These strategies leverage psychological expectations, enhancing your chances of winning.

Why This Strategy Works

To illustrate why these strategies are effective, consider this scenario: If you call ‘Rock’ and your opponent calls ‘Scissors’ (resulting in a win for you), they may anticipate that you will call ‘Rock’ again. In response, they might choose ‘Paper’ to counter your expected move. Thus, calling ‘Scissors’ next increases your chances of winning.

Alternatively, if you lose after calling ‘Rock’ against an opponent’s ‘Paper’, they are likely to stick with ‘Paper’. Therefore, calling ‘Scissors’ in the next round could yield a better outcome.

These strategies, grounded in empirical research, provide an edge based on human psychology. However, if both players are aware of these tactics, the game becomes more complex, and random selection may become the preferable strategy. But if you possess this knowledge while your opponent does not, it could significantly increase your chances of success. Best of luck!

Credit: Wang et al. (original research paper), Hannah Fry (analysis), and Brady Haran (presentation).

I hope you found this exploration of Rock-Paper-Scissors insightful. If you enjoyed this content, consider supporting me by clapping, following, or subscribing.

For further reading, check out topics like "What Really Happens When You Divide By Zero?" and "The Fascinating Reason Why Temperature Has No Upper Limit."

You can view the original essay here.